Chapter 6 – Coastal Revetments for Wave Attack

From ‘Highways in the Coastal Environment: Second Edition’ by FHWA

Chapter 6 – Coastal Revetments for Wave Attack

6.1 – 6.2 – 6.3 – 6.4

This section addresses the design of revetments on embankments for protection from wave attack. The design of an earthen highway embankment is primarily a geotechnical engineering problem with rock or rip-rap revetments sometimes employed as slope protection. Revetments can be used for protection from four different types of hydraulic situations: direct rainfall impacts, overland flow, stream or river currents, and waves. This section addresses only wave attack.

HEC-11 (Brown and Clyde 1989) provides procedures for the design of riprap revetments for channel bank protection on larger streams and rivers where the active force of the flowing water exceeds the bank material’s ability to resist movement. Flow in a stream or river is unidirectional and typically aligned parallel to the banks. Waves produce oscillatory velocities and accelerations that can be in almost any direction on a revetment. HEC-11 recommends Hudson’s equation to estimate stone size for revetments subject to wave action.

This section recommends an approach based on determining a design wave and using Hudson’s equation to size the stones in the outer layer of a rock revetment. This approach can lead to designs with larger stones and narrower stone gradations than designs for non-wave situations. The difference is due to the higher forces caused by waves. Situations where riverine and wave flows are significant, the design engineer should consider both design approaches and develop a conservative design.

6.1 Types of Revetments and Seawalls

Figure 6.1 shows a revetment along a bay shoreline designed to protect a local road from erosion by waves during storms. This design has a stone revetment extending from below the water surface up to a sheet pile wall and pile cap near the roadway shoulder. Storm surges can exceed the pavement elevation here.

The distinction between revetments, seawalls, and bulkheads is one of functional purpose (USACE 1984). Revetments are layers of protection on the top of a sloped surface to protect the underlying soil. Seawalls are walls designed to protect against large wave forces. Bulkheads are designed primarily to retain the soil behind a vertical wall in locations with less wave action. Design issues such as tie-backs, depth of sheets are primarily controlled by geotechnical issues. Given the relationship between wave height and fetch (distance across the water body) Figure 6.2 provides a conceptual distinction between the three types of coastal protection. Bulkheads are most common where fetches and wave heights are very small. Seawalls are most common where fetches and wave heights are very large. Revetments are often common in intermediate situations such as on bay or lake shorelines.

Seawalls can be rigid structures or rubble-mound structures specifically designed to withstand large waves. Two very large, rigid, concrete seawalls with recurved tops to minimize overtopping are the Galveston Seawall (Figure 6.3) and San Francisco’s Great Highway Seawall (Figure 6.4). Such massive structures are not commonly constructed in the US. Vertical sheet pile seawalls with concrete caps are common but require extensive marine structural design. A more common seawall design type in the United States is a rubble-mound that looks very much like a revetment with larger stones to withstand the design wave height. Thus, the two terms, seawalls and revetments, can be used interchangeably with the former typically used for the larger wave environments. Figure 6.5, Figure 6.6, Figure 6.7, and Figure 6.8 are examples of rubble-mound seawalls protecting coastal roads exposed to open-coast storm waves.

Figure 6.1. A revetment protecting a coastal highway (Bayfront Road, Mobile, Alabama, 2001)

Figure 6.2. Types of shore protection walls.

Figure 6.3. Galveston Seawall (Seawall Blvd.1983)

Figure 6.4. San Francisco’s Great Highway Seawall (California Highway 35, 1991)

Figure 6.5. Seawall protecting a coastal highway. (Venice, Florida)

Figure 6.6. Seawall protecting a coastal highway (Pacific Coast Highway, Pacific Palisades, California)

Figure 6.7. Seawall protecting a coastal highway (Florida Highway A1A, Flagler Beach)

Figure 6.8. Seawall protecting a coastal highway (US Highway 101, Curry County, Oregon)

Figure 6.9 and Figure 6.10 (as well as Figure 6.1) are examples of rubble-mound revetments protecting highways along coastal bays. Revetments are common on bay or lake shorelines where design waves are short-period, fetch-limited, locally-generated storm waves.

Figure 6.9. Revetment protecting a highway along a bay shoreline (Florida Highway 60, Tampa Bay)

Figure 6.10. Revetment protecting a highway along a bay shoreline (Washington State Route 105, Willapa Bay)

Revetments have been criticized for a variety of reasons, including their aesthetics. Figure 6.11 and Figure 6.12 show two different types of protection designed for local roads that were threatened by bluff erosion. Figure 6.11 shows a rock revetment and Figure 6.12 shows a concrete wall that has been designed to look much like the natural bluff. The engineered seawall is in the middle of the Figure 6.12 image. The more aesthetically pleasing seawall (Figure 6.12) was designed more recently than the rock revetment. This is an example of the evolving nature of seawall design in the United States.

Figure 6.11. Seawall protecting a local road (West Cliff Drive, Santa Cruz, California)

Figure 6.12. Concrete seawall designed to look like the natural rock formation built on an eroding sea cliff to protect a local road (East Cliff Drive, Santa Cruz, California)

6.2 Hudson’s Equation for Armor Stone Size

A well-designed and constructed rubble-mound revetment can protect embankments from waves. The underlying philosophy of the rubble-mound is that a pile of stones is efficient at absorbing wave energy and robust in design in that damage is often not catastrophic. It also can be relatively inexpensive. Some of the oldest coastal structures in the world are rubble-mounds. They have the inherent ability to survive storms in excess of their design storm. In the words of an old advertisement for a brand of watches, rubble-mound revetments “can take a licking and keep on ticking.” This ability to continue to provide some function even after experiencing storms that are more severe than their design storm is valuable in a coastal environment where costs often preclude selection of extremely rare design storms.

Hudson’s equation (USACE 1984) provides a basis for estimating the required stone size in a sloped revetment. The required median weight for the outer, or armor layer, stones is:

 (6.1)

where:

W50	=	median weight of armor stone
wr	=	unit weight of stone (~165 lb/ft3)
H	=	design wave height
KD	=	empirical coefficient (=2.2 for rip-rap gradations)
Sr	=	specific gravity of stone (~2.65)
θ	=	slope

Hudson’s equation accounts for the most important variables including design wave height, different structure slopes, different stone densities and angularities. Steeper slopes require larger stones. However, the range of recommended slopes here is up to 2:1 (horizontal:vertical). Note that, by definition, the cotq=2 for a 2:1 slope and cotq=3 for a 3:1 slope, etc. Revetment structure slopes greater than 1½:1 (horizontal:vertical) are not recommended (USACE 1984).

The empirical coefficient in Hudson’s Equation, K_D, is based on laboratory tests and varies to include the effect of stone angularity/roundness, number of layers of armor stone, distribution of individual stone sizes about the median size, and interlocking characteristics. The value suggested here, K_D = 2.2, is for a layer of rough-angular quarrystone at least two stones thick. The stones have a gradation of weights that varies between 0.125 W₅₀ < W < 4W₅₀. Other values of K_D for other situations, including artificial concrete armor units, are discussed in USACE (1984) and USACE (2002).

For typical conditions of specific gravity of stone (S_r=2.65 for granite) and unit weight of stone (w_r=165 lb/ft3), with the empirical coefficient set to K_D=2.2, Equation 6.1 can be written as:

 (6.2)

where:

W50	=	median weight of armor stone (lbs)
H	=	design wave height (feet)
θ	=	slope

Figure 6.13 shows a typical revetment design cross-section. The armor layer stones have a median weight given by Hudson’s equation. One component of the design is a filter cloth geotextile or composite geotextile/geogrid between the rocks and the underlying soil. A geotextile that provides rapid transfer of water through the material while holding soil particles and is strong enough to survive the construction process without puncturing by the overlying rocks is recommended. The modern use of a plastic grid integrally welded to the geotextile can provide some additional strength to bridge soft underlying soils. The geotextile should be designed to not allow the rocks to slide down the surface. The use of an underlayer of stones between the armor layer and the geotextile/grid is common except when the stone size is less than 200 lb. The underlayer should have a median weight no smaller than one-tenth that of the armor layer stones (USACE 1984). Smaller underlayer stones can be pulled out between the gaps of the armor stones.

Figure 6.13. Typical coastal revetment design cross-section

6.3 Design Wave Heights for Revetment Design

The estimate of the required armor stone size from Hudson’s equation is sensitive to wave height. The proper wave height for Hudson’s equation above for coastal revetment design is either the depth-limited maximum wave height or the average of the highest 10% of all wave heights in the design sea-state () whichever is lesser (USACE 1984).

This recommendation is based on interpretation considering the origin of the equation. Hudson’s equation was originally derived based on monochromatic laboratory tests. Thus, the proper selection of a corresponding wave height statistic from an irregular sea-state is not obvious. Experience has found that the use of the significant wave height, H_s, in Hudson’s equation is not conservative and can lead to undesired levels of damage to the revetment.

Some researchers have suggested that the proper irregular wave height statistic for use in Hudson’s equation is . To be conservative, some engineers use the average of the highest 5% of all wave heights in the design sea-state (). The relationships (see Table 4.1) between significant wave height and these other statistics are = 1.27 H_s and = 1.38 H_s.

Coastal revetments are often located where the design sea-state is depth-limited, i.e. the depths are so shallow immediately offshore of the location of the revetment that the storm waves have broken and the largest waves are on flat offshore slopes,

 (6.3)

where:

Hb	=	maximum breaking wave height
ds	=	design depth at the toe of the structure

To account for the distance over which waves travel as they break, a depth some distance offshore of the toe (say one wavelength) sometimes is used in Equation 6.3. For non flat slopes see USACE (1984) and USACE (2002).

A depth-limited design wave height used in Hudson’s equation should account for any long-term erosion that may change the depths immediately offshore. The construction of a revetment, while it protects the upland, does not address the underlying cause of erosion. The depths at the toe of the revetment will likely increase if the erosion process continues. The presence of a revetment or seawall can increase the vertical erosion at its base. The revetment or seawall does not allow the material in the bluff to naturally nourish the beach.

Hudson’s equation has no factor-of-safety. Hudson established the K_D values such that there was some small level of damage to the structure. The damage level was defined as the level where 5% of the rocks on the revetment structure armor layer face moved. Thus, it is entirely appropriate for some conservatism or factor of safety to be added to the design process based on engineering judgment. The factor of safety could be included through the selection of a conservative design wave height used (such as  in Hudson’s equation or it could be through an increase in the specified design median rock weight.

Applications of Hudson’s equation in situations with a design significant wave height of H = 5 feet or less have performed well. This range of design wave heights encompasses many coastal revetments along highway embankments. When design wave heights get very large and the design water depths get very large, problems with the performance of rubble-mound structures can occur. These problems relate in part to wave groupiness (back to back large waves) , design sea-state specification, constructability and other issues. Seawalls with design wave heights much greater than H=5 feet require more judgment and more experience and input from a trained, experienced coastal engineer. Other details about the design of rubble-mound revetments are discussed in the Coastal Engineering Manual (USACE 2002).

One alternative to the two-layer design of Figure 6.13, is a “dynamic revetment” (or “berm revetment”) which contains a significantly larger volume of smaller stones with a wider gradation. A dynamic revetment allows the stones to move in response to storm waves into an equilibrium shape much like a cobble or sand beach.

An alternative to the use of extremely large stones in the armor layer is to use concrete armor units. These typically are lighter since they interlock better than quarrystone and thus have higher K_D values. They can be cast on site. There are a number of shapes of artificial concrete armor units including several patented shapes requiring the payment of license fees.

6.4 Practical Issues for Coastal Revetment Design

The stone gradations recommended above for coastal revetments are much narrower than those typically used for highways. For example, FHWA’s Standard Specifications for Class 5 rip-rap call for a median weight of W₅₀ = 770 lbs, with 10% of the stones weighing 0 to 55 lbs., 40% weighing 55 to 770 lbs, 30% weighing 770 to 1540 lbs, and 20% weighing 1540 to 2200 lbs (USDOT 2003).

A footnote to the FHWA specification table says “furnish spalls and rock fragments graded to provide a stable dense mass.” However, the gradation recommended above for Hudson’s equation for coastal revetment for the same median weight of W₅₀ = 770 lbs, calls for all stones to weigh between 100 and 3000 lbs. Thus, the recommended coastal revetment gradation precludes the smaller stones and allows for some larger stones. These smaller stones are typically not included in coastal revetments because of their tendency to move in response to wave action. If there is a potential for the smaller stones that are removed from the revetment during storms causing other damage as projectiles, then the narrower gradation, without the smaller rocks, should be required. This typically results in higher unit costs for the stone.

There are five typical failure mechanisms for coastal revetments:

1. inadequate armor layer design for wave action,
2. inadequate under layer,
3. flanking,
4. toe scour, and
5. overtopping splash.

A revetment’s strength depends on the underlying soil. If wave action can remove that soil via any mechanism, the revetment will collapse. Each of the four typical failure mechanisms involves failure to protect that underlying soil. Each can be prevented by careful design by an experienced engineer.

Figure 6.14 shows a failed attempt to protect an embankment. The slope protection used concrete slab panels. The concrete panels were available from some other project and were set on the surface of the eroding bluff. Although the panels were heavy enough to withstand the wave action itself, wave action during storms likely pulled, or pumped, the underlying soil out from between the gaps in the slabs. Consequently, the panels collapsed. The second photograph shows the panels after collapse. A rock revetment was subsequently placed farther back on the bluff. The original panel design did not adequately protect the underlying soil and did not have the flexibility of a rubble-mound revetment.

Figure 6.14. Example of a failed attempt at embankment protection (USACE archives photo)

Hudson’s equation can usually be used to select the stone size in the outer layer of a revetment subjected to wave attack and it was specifically developed for that situation. However, careful engineering judgment based on experience should be used when the design cross-section varies from that in Figure 6.13. Figure 6.15 shows a revetment protecting a highway that has a small, vertical bulkhead with stones on the seaward side and an almost flat stone section landward. This cross-section design essentially “trips” breaking waves when storm surge raises the water level and begins to inundate the highway. Thus, breaking waves can plunge directly on the stones and move them onto and across the roadway during major storms. For very mild slopes, Hudson’s equation estimates very small armor stone and adjustments may be needed. A larger stone weight would prevent this type of failure.

Figure 6.15. A revetment with rocks that are too small to withstand wave attack

Flanking occurs when adjacent, unprotected shorelines continue to recede. Erosion at the end of the wall allows wave action to remove the soil from behind the wall starting at the ends, then progressing along the walls it fails. Flanking can be avoided by extending the revetment or wall to meet an existing revetment or a wall or natural rock outcropping, or by using a return wall. A return wall is aligned perpendicular to the shoreline. The length of the return wall should exceed the expected long-term and storm-induced recession of the adjacent shorelines.

Vertical scour at the toe of a revetment or seawall can cause the underlying soil to be exposed to waves. One solution to toe scour problems is shown in the recommended revetment cross-section in Figure 6.13. A significant volume of stones is placed at the toe. This toe is designed to collapse into any toe scour hole that develops without loss of the stones on the slope. For very erosive areas, more stones can be used in the toe.

Overtopping splash at the top of a revetment or seawall can also lead to failure by exposing the underlying soil to waves. If the wall does not extend to a high enough elevation, waves will overtop the wall. Figure 6.16 shows indications of overtopping splash damage at the top of rock seawall.

Figure 6.16. An example of splash damage behind seawall

A solution to overtopping splash problems is to provide a splash apron as is shown in the revetment cross-section in Figure 6.13. The rocks extend some distance back from the break in slope. The width of the splash apron varies depending on the severity of the expected overtopping. A minimal splash apron width is 5 to 10 feet.

The elevation of the top of the revetment in Figure 6.13 was based on the elevation of the top of an existing embankment. It was assumed that wave runup would allow some limited overtopping at the design conditions. The splash apron was thus included. For situations where the embankment elevation is much higher than the expected level of wave runup during design conditions, a decision regarding the height of the revetment is required. The height of wave runup (R_u) is shown in Figure 6.17. It can be estimated using:

 with a maximum of 3.2 r (6.4)

where:

Ru,2%	=	runup level exceeded by 2% of the runups in an irregular sea
Hs	=	significant wave height near the toe of slope
r	=	a roughness coefficient (r = 0.55 for the stone revetments)
ξop	=	the surf similarity parameter as defined below

 (6.5)

where:

θ	=	angle of slope of structure (see Figure 6.17)
Hs	=	significant wave height
Tp	=	wave period, peak period
g	=	acceleration of gravity

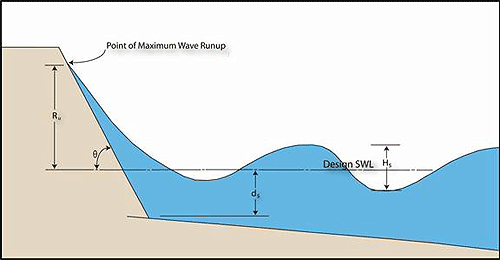
Figure 6.17. Wave runup definition sketch

The level given by Equation 6.4 is for the 2% runup level. This runup level is defined as the runup level exceeded by 2% of the incoming waves. Thus, 2% of the waves will run up higher than this level. The roughness coefficient (r) accounts for the roughness of the surface of the revetment with r = 1 for smooth slopes. For rock revetments such as shown in Figure 6.11, the recommended value for r is 0.55. For r=0.55, Equation 6.4 has an upper limit of 3.2r = 1.76. Thus, the 2% level of runup is < 1.76H_s. Equation 6.4 is adapted from a methodology developed by Van der Meer and summarized by Pilarczyk (1999). More detail including other structure geometries can be found in that reference.

Wave overtopping of revetments and seawalls occurs when runup exceeds the top or crest of the structure. Building seawalls high enough to completely prevent overtopping is often unacceptable because of aesthetics and costs. Wave overtopping onto coastal roads is fairly common in some parts of the country. Two aspects of overtopping of interest to the design engineer are the time-averaged volumetric rate of overtopping and the intensity or force of a single wave overtopping event. Accurately estimating volumetric overtopping rates can be vital to design of seawall crest elevations if inland flooding is caused. Unfortunately, accurately estimating overtopping rates can be very difficult for many situations and input to the design team from a trained coastal engineer is likely appropriate. Guidance on estimating overtopping can be found in Goda (1985) and USACE (2002).

A commonly proposed alternative to rubble mound revetments is a concrete block revetment. Some of these have some physical interlocking between individual blocks and others do not. The performance of interlocking blocks in severe coastal environments has not been good. One problem is that minor damage can lead to failure of a large portion of the revetment. Two examples are shown in Figure 6.18 and Figure 6.19. The failed revetment in Figure 6.18 has been covered by a sand beach through beach nourishment (see Figure 7.16 ). The failed revetment in Figure 6.19 has been replaced by a sand beach through beach nourishment and stabilized by offshore segmented breakwaters (see Figure 7.18). Problems with concrete block revetments in coastal situations often develop at the ends of the revetment where the blocks abut a more rigid structure. Even a small amount of settlement can affect the aesthetics of block revetments.

Figure 6.18. Example of rigid concrete-block revetment failure (Florida Highway A1A, Delray Beach, circa 1972; University of Florida and USACE archive photos)

Figure 6.19. Example of failed block revetment (Louisiana Highway 87, circa 1980, USACE archives photos)

Another commonly proposed alternative to rubble mound revetments are rigid concrete panel designs. Performance of rigid concrete panels in severe coastal environments also has not been good. A concrete panel revetment on a bridge approach that suffered damage in a hurricane is shown in Figure 6.20. The underlying soil was not adequately protected from wave attack. Neither interlocking blocks nor concrete panels match the performance and flexibility of stone revetments. The Florida DOT does not allow rigid revetments in wave situations.

Figure 6.20. Example of rigid revetment failure on a coastal highway bridge approach

Other revetment systems include articulated concrete mats, flexible rock-filled marine mattresses, gabions, and sand-filled geotextile tubes or bags. Articulated concrete mats have concrete blocks interconnected by strong cables. The size and weight of the blocks are a function of the wave height, slope, currents, etc. Proper installation requires adequate filtration material and secure anchoring at the top of the slope. The toe is sometimes unsecured to allow it to settle (scour). Flexible rock-filled marine mattresses are used as foundations and for scour control underneath marine structures; but they are not generally recommended for slope protection by themselves. Gabions are rock-filled “baskets” composed of steel wire or polypropylene grid which are stacked for embankment protection. Their use in energetic coastal environments, where wave heights may routinely exceed 1 to 3 feet, is not generally recommended. Sand-filled geotextile containers (tubes or bags) are typically only used for temporary, interim embankment protection in the coastal zone. Where used, they are best buried within the existing grade and become exposed only during storm erosion (an example is illustrated in Figure 7.3). The structures are prone to damage or failure by vandalism, rolling, and natural deterioration when exposed.